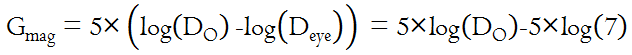Ok so we were supposed to be talking about your telescope so let's get back to that. We've already worked out the brightness increase we get from the scope as GL = (DO/Deye)², so all we need to do is take 2.5×log(GL) and we have the brightness increase of the scope in terms of magnitudes, so it's just Gmag = 2.5×log((DO/Deye)²).
We can take advantage of the logarithm in the equation to simplify it, by making use of the fact that log(x²) = 2×log(x). So then:
When you divide by a number you subtract its logarithm, so subtracting the log of Deye from DO , then substituting 7mm for Deye , we get:
Since log(7) is about 0.8, then 5×0.8 = 4 so our equation for the gain in star magnitude is
This represents how many more magnitudes the scope lets me see, over and above what my eye alone can see. Then to find the faintest magnitude I can see in the scope, we simply add Gmag to the faintest magnitude our eye can see, magnitude 6. This is the magnitude limit of the scope, Lmag:
We can take advantage of the logarithm in the equation to simplify it, by making use of the fact that log(x²) = 2×log(x). So then:
When you divide by a number you subtract its logarithm, so subtracting the log of Deye from DO , then substituting 7mm for Deye , we get:
Since log(7) is about 0.8, then 5×0.8 = 4 so our equation for the gain in star magnitude is
This represents how many more magnitudes the scope lets me see, over and above what my eye alone can see. Then to find the faintest magnitude I can see in the scope, we simply add Gmag to the faintest magnitude our eye can see, magnitude 6. This is the magnitude limit of the scope, Lmag:
Which simplifies down to our final equation for the magnitude limit Lmag of the scope
By the way did you notice through all this, that the magnitude limit of the scope — the faintest star I can see in the scope — depends only on the diameter of the objective? Interesting result, isn't it?
You Like This Topic? Then Share It With Your Friends!